1339. Maximum Product of Splitted Binary Tree --------M ~~！！！！~

 Given a binary tree root. Split the binary tree into two subtrees by removing 1 edge such that the product of the sums of the subtrees are maximized.

Since the answer may be too large, return it modulo 10^9 + 7.

 

Example 1:

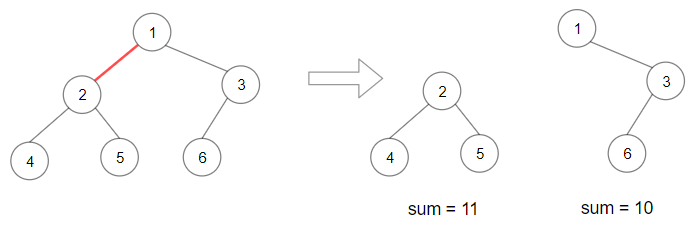
Input: root = [1,2,3,4,5,6]
Output: 110
Explanation: Remove the red edge and get 2 binary trees with sum 11 and 10. Their product is 110 (11*10)

Example 2:

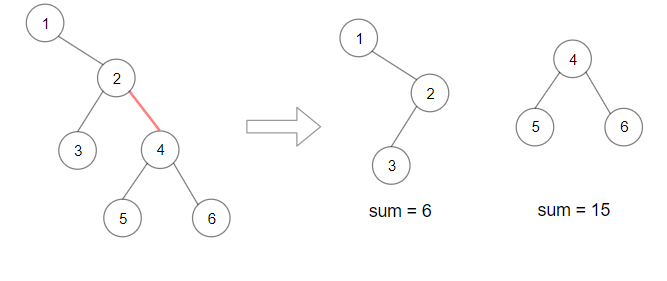
Input: root = [1,null,2,3,4,null,null,5,6]
Output: 90
Explanation:  Remove the red edge and get 2 binary trees with sum 15 and 6.Their product is 90 (15*6)

Example 3:

Input: root = [2,3,9,10,7,8,6,5,4,11,1]
Output: 1025

Example 4:

Input: root = [1,1]
Output: 1

 

Constraints:

• Each tree has at most 50000 nodes and at least 2 nodes.
• Each node's value is between [1, 10000].

A:

递归，没什么好说的。

需要注意的的是， int 类型的相乘， 一定要用long ,防止overflow.

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    int maxProduct(TreeNode* root) {
        int modulo  = pow(10,9)+7;  // INT is 32 bit
        vector<int> sums;
        int total = helper(root, sums, true);//long is 64 bit on 64 bit OS,(but 32 on linux 32-bit)
        long res = INT_MIN;
        for(auto v : sums){
            res = max(res, (long(v) * (total -v)));
        }
        return int(res%modulo);
    }
private:
    int helper(TreeNode* root, vector<int> & S, bool isRoot){
        if(not root)
            return 0;
        int curSum = root->val + helper(root->left,S,false) + helper(root->right,S,false);
        if(not isRoot)
            S.push_back(curSum);        
        return curSum;
    }
};

ERRORS:

肏，这里犯了一个很傻的错误。

自己一开始都设成int 类型，因此为了不overflow, 就

        for(auto v : sums){
            res = max(res, (long(v) * (total -v))%modulo);
        }

这样，结果是不对的~~~~~~~~~~~~~